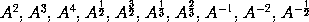Sir Isaac Newton, The Mathematical Principles of Natural Philosophy (Philosophiae Naturalis Principia Mathematica) Book Two. Translated by Andrew Motte (1729).
LEMMA II
I call any quantity a Genitum, which is not made by addition or subduction of divers parts, but is generated or produced in arithmetic by the multiplication, division, or extraction of the root of any terms whatsoever; in geometry by the invention of contents and sides, or of the extreams and means of proportionals. Quantities of this kind are products, quotients, roots, rectangles, squares, cubes, square and cubic sides and the like. These quantities I here consider as variable and indetermined, and increasing or decreasing as it were by a perpetual motion or flux; and I understand their momentaneous increments or decrements by the name of Moments; so that the increments may be esteem’d as added, or affirmative moments; and the decrements as subducted, or negative ones. But take care not to look upon finite particles as such. Finite particles are not moments, but the very quantities generated by the moments. We are to conceive them as the just nascent principles of finite magnitudes. Nor do we in this Lemma regard the magnitude of the moments, but their first proportion as nascent. It will be the same thing, if, instead of moments, we use either the Velocities of the increments and decrements (which may also be called the motions, mutations, and fluxions of quantities) or any finite quantities proportional to those velocities. The coefficient of any generating side is the quantity which arises by applying the Genitum to that side.
Wherefore the sense of the Lemma is, that if the moments of any quantities A, B, C, &c. increasing or decreasing by a perpetual flux, or the velocities of the mutations which are proportional to them, be called a, b, c, &c. the moment or mutation of the generated rectangle AB will be aB + bA; the moment of the generated content ABC will be aBC + bAC + cAB: and the moments of the generated powers
will be
respectively. And in general, that the moment of any power  will be
will be 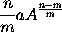 Also that the moment of the generated quantity A²B will be 2aAB + bA²; the moment of the generated quantity
Also that the moment of the generated quantity A²B will be 2aAB + bA²; the moment of the generated quantity 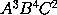 will be
will be  ; and the moment of the generated quantity
; and the moment of the generated quantity 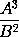 or
or 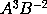 will be
will be 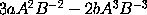 ; and so on. The Lemma is thus demonstrated.
; and so on. The Lemma is thus demonstrated.
Case 1. Any rectangle as AB augmented by a perpetual flux, when, as yet, there wanted of the sides A and B half their moments 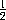 a and
a and 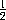 b, was A –
b, was A – 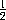 a into B –
a into B – 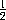 b, or AB –
b, or AB – 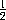 aB –
aB – 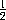 Ba +
Ba + 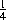 ab; but as soon as the sides A and Bare augmented by the other half moments; the rectangle becomes A +
ab; but as soon as the sides A and Bare augmented by the other half moments; the rectangle becomes A + 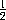 a into B +
a into B + 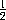 b, or AB +
b, or AB + 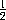 aB +
aB + 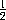 Ba +
Ba + 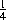 ab. From this rectangle subduct the former rectangle, and there will remain the excess aB + bA. Therefore with the whole increments a and b of the sides, the increment aB + bA of the rectangle is generated. Q.E.D.
ab. From this rectangle subduct the former rectangle, and there will remain the excess aB + bA. Therefore with the whole increments a and b of the sides, the increment aB + bA of the rectangle is generated. Q.E.D.
Case 2. Suppose AB always equal to G, and then the moment of the content ABC or GC (by Case 1.) will be gC + cG, that is, (putting AB and aB + bA for G and g) aBC + bAC + cAB. And the reasoning is the same for contents, under never so many sides. Q.E.D.
Case 3. Suppose the sides A, B, and C, to be always equal among themselves; and the moment aB + bA, of A², that is, of the rectangle AB, will be 2aA; and the moment aBC + bAC + cAB of A³, that is, of the contentABC, will be 3aA². And by the same reasoning the moment of any power 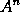 is
is 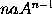 . Q.E.D.
. Q.E.D.
Case 4. Therefore since 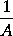 into A is 1, the moment of
into A is 1, the moment of 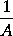 drawn into A, together with
drawn into A, together with 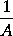 drawn into a, will be the moment of 1, that is, nothing. Therefore the moment of
drawn into a, will be the moment of 1, that is, nothing. Therefore the moment of 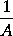 or of
or of 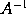 is
is 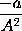 . And generally, since
. And generally, since 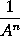 into
into 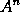 is 1, the moment of
is 1, the moment of 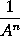 drawn into
drawn into 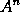 together with
together with 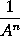 into
into 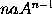 will be nothing. And therefore the moment of
will be nothing. And therefore the moment of 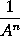 or
or 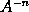 will be
will be 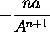 . Q.E.D.
. Q.E.D.
Case 5. And since 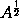 into
into 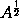 is A, the moment of
is A, the moment of 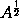 drawn into 2
drawn into 2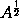 will be a, (by Case 3:) and therefore the moment of
will be a, (by Case 3:) and therefore the moment of 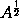 will be
will be  or
or 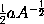 . And generally putting
. And generally putting 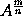 equal to B, then
equal to B, then 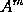 will be equal to
will be equal to 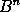 , and therefore
, and therefore 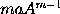 equal to
equal to 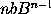 , and
, and 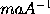 equal to
equal to 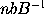 or
or 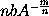 ; and therefore
; and therefore 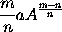 is equal to b, that is, equal to the moment of
is equal to b, that is, equal to the moment of 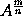 . Q.E.D.
. Q.E.D.
Case 6. Therefore the moment of any generated quantity 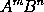 is the moment of
is the moment of 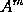 drawn into
drawn into 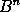 , together with the moment of
, together with the moment of 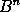 drawn into
drawn into 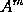 , that is,
, that is, 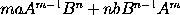 ; and that whether the indices m and n of the powers be whole numbers or fractions, affirmative or negative. And the reasoning is the same for contents under more powers. Q.E.D.
; and that whether the indices m and n of the powers be whole numbers or fractions, affirmative or negative. And the reasoning is the same for contents under more powers. Q.E.D.
Cor. 1 Hence in quantities continually proportional, if one term is given, the moments of the rest of the terms will be as the same terms multiplied by the number of intervals between them and the given term. Let A, B, C, D,E, F, be continually proportional; then if the term C is given, the moments of the rest of the terms will be among themselves, as -2A, -B, D, 2E, 3F.
Cor. 2 And if in four proportionals the two means are given, the moments of the extremes will be as those extremes. The same is to be understood of the sides of any given rectangle.
Cor. 3. And if the sum or difference of two squares is given, the moments of the sides will be reciprocally as the sides.
Scholium
Links:
D.R. Wilkins (dwilkins@maths.tcd.ie) School of Mathematics Trinity College, Dublin